Confidence Interval Calculator: A Tool for Statistical Analysis

You will learn how to determine confidence intervals accurately using a simple, user-friendly confidence interval calculator.

Introduction

Welcome to an insightful journey into statistical analysis, where we explore the vital role of confidence intervals and the utility of the confidence interval calculator.

This essential statistical tool quantifies uncertainty in data interpretation, providing a range where the true value of a parameter likely lies. With the confidence interval calculator, this complex task becomes accessible to everyone, from seasoned statisticians to those new to data analysis.

This guide delves into the nuances of confidence intervals, their practical applications, and how to utilize the calculator for accurate and reliable statistical analysis.

Highlights

Discover the ease of calculating accurate confidence intervals with our intuitive calculator.
Learn the importance of confidence intervals in making informed statistical conclusions.
Understand the impact of sample size and standard deviation in confidence interval calculations.
Explore practical applications of confidence intervals in various research scenarios.
Gain insights into avoiding common mistakes while interpreting confidence intervals.

Understanding Confidence Intervals

In statistics and data analysis, confidence intervals play a pivotal role. They are not merely numbers but a profound reflection of the reliability and precision inherent in statistical estimates. This section delves into the essence of confidence intervals and elucidates why they are indispensable in data analysis.

What Are Confidence Intervals?

A confidence interval provides a range of values, estimated from the data, that is likely to contain the true value of an unknown population parameter. It is expressed with a certain level of confidence, commonly 95% or 99%. If we were to take multiple samples and compute the confidence interval for each, we would expect the true population parameter to fall within these intervals in 95% or 99% of the cases, respectively.

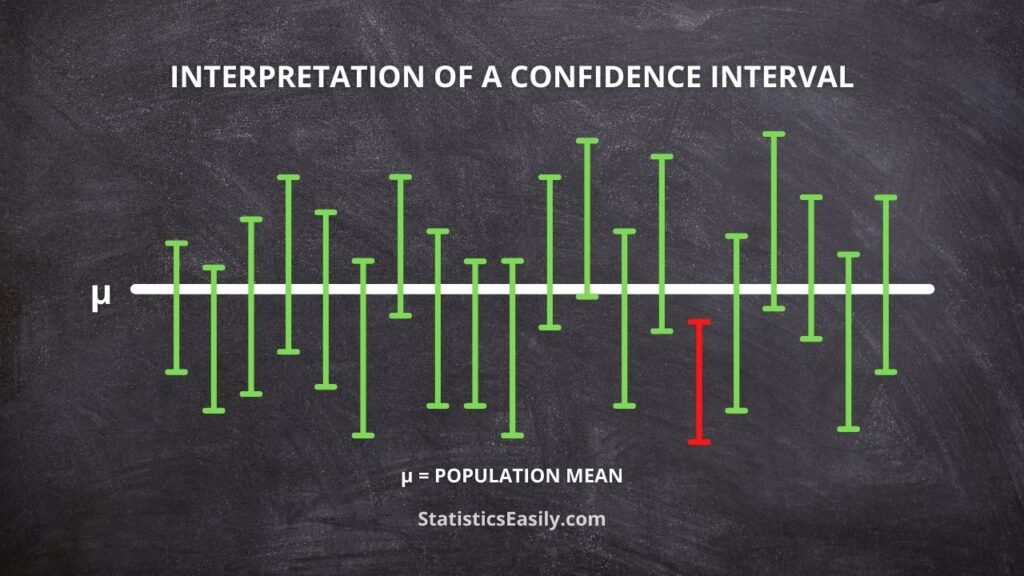
The Significance of Confidence Intervals in Data Analysis

Why are confidence intervals so crucial? They offer a window into the precision of our estimates. By presenting a range rather than a single estimate, confidence intervals acknowledge the inherent variability in data and measure the uncertainty around the estimated value. This is vital for informed decision-making, particularly in scientific research, policy development, and business strategy, where data reliability is paramount.

A Foundation of Trustworthy Analysis

At the heart of confidence intervals is the pursuit of truth — a commitment to presenting data analysis that is accurate and reflective of reality’s complexity. They empower us to make predictions and decisions grounded in a deeper understanding of data, steering us away from potentially misleading conclusions based on single-point estimates. In this way, confidence intervals are more than just a statistical tool; they are a testament to our dedication to rigorous and ethical data analysis.

Confidence Interval Formula

x ± Z × σ / √n

x = Sample Mean

Z = Z-score (Critical value from the standard normal distribution)

σ = Population Standard Deviation

n = Sample Size

The Role of the Confidence Interval Calculator

The confidence interval calculator is a straightforward tool that aids in simplifying the process of calculating confidence intervals. It offers a user-friendly interface where users can input key statistical parameters such as sample mean, standard deviation, and sample size. The calculator then quickly computes the confidence interval, providing accurate and immediate results.

This tool is valuable for a wide range of users, from seasoned statisticians who require a quick means to validate their calculations to beginners who are just starting to understand the concept of confidence intervals. Its primary utility lies in its ability to deliver precise calculations essential for reliable statistical analysis. Overall, the confidence interval calculator is a practical and efficient resource in the toolkit of anyone involved in statistical data analysis.

Step-by-Step Guide: Confidence Interval Calculator

This section provides a detailed, step-by-step guide to effectively use the calculator, ensuring that even those new to statistics can confidently calculate confidence intervals.

Step 1: Entering the Sample Mean: Enter the sample mean in the calculator. The sample mean, denoted as Sample Mean in the calculator, is the average of your data set. It’s the central value around which your confidence interval will be calculated.

Step 2: Inputting the Standard Deviation: The next step involves entering the standard deviation of your data set. The Standard Deviation field in the calculator requires this input. The standard deviation reflects the dispersion of your data from the mean, a critical factor in determining the width of your confidence interval.

Step 3: Specifying the Sample Size: The Sample Size input is crucial as it influences the accuracy of your interval. A larger sample size generally leads to a more precise confidence interval. Input the number of observations or data points in your sample here.

Step 4: Selecting the Significance Level: Choose your desired confidence level from the Significance Level dropdown. Standard options include 90%, 95%, and 99% levels, translating to significance levels of 0.1, 0.05, and 0.01, respectively. This choice dictates how confident you want to be about the interval containing the true population parameter.

Step 5: Calculating the Confidence Interval: After filling in the necessary data, click the Calculate button. The calculator will apply the Z-score corresponding to your chosen confidence level to compute the margin of error and, subsequently, the confidence interval.

Step 6: Interpreting the Results: The calculated interval will be displayed under the result section. Given your specified confidence level, it provides you with a range where the true population parameter is likely to be found.

Confidence Interval Calculator

Practical Applications of Confidence Intervals

This section explores how confidence intervals are pivotal in diverse areas, highlighting their practicality and importance.

Application in Scientific Research: In scientific studies, confidence intervals are crucial for estimating parameters like mean blood pressure, average growth rate, or medication effectiveness. They provide a range within which researchers are confident the true value lies based on their sample data. This helps understand the variability inherent in biological processes and make generalizations from sample data to a larger population.

Use in Market Research: Market researchers rely on confidence intervals to understand consumer behavior and preferences. For instance, when determining the average amount a customer is willing to pay for a product, confidence intervals provide a range for this average, offering insights into pricing strategies. They aid businesses in making data-driven decisions by quantifying the uncertainty in survey results.

Impact on Policy Development: Policymakers often use confidence intervals to inform decisions. For example, understanding the confidence interval of a disease prevalence rate in public health can guide resource allocation and intervention strategies. These intervals help policymakers assess the reliability of the data they use to make critical decisions affecting communities.

Application in Quality Control: In manufacturing and quality assurance, confidence intervals are used to determine whether a process is consistent and whether products meet the required standards. By calculating the confidence interval of a quality metric, such as the diameter of a manufactured part, engineers can assess if the production process is under control and meets the specified tolerance levels.

Role in Financial Analysis: Confidence intervals are also integral in financial analysis, such as forecasting future stock prices or evaluating investment risks. They give investors and financial analysts a range in which they can expect returns, helping in risk assessment and portfolio management.

Common Misconceptions and Mistakes

This section aims to clarify these misunderstandings and provide guidance on avoiding common pitfalls.

Misconception 1: Confidence Intervals Provide Absolute Certainty: A common error is to interpret confidence intervals as providing absolute certainty about the range of the parameter. It’s crucial to understand that these intervals reflect the probability of the parameter falling within a range based on the sample data. They are not guarantees but probabilistic indications.

Misconception 2: Overlooking the Impact of Sample Size: Often, the sample size’s significance in determining the confidence interval’s width is underestimated. A larger sample size generally results in a narrower confidence interval, offering more precision. Considering the sample size’s impact when interpreting confidence intervals is important.

Mistake 1: Misinterpreting the Confidence Level: There’s a frequent misunderstanding about what the confidence level (e.g., 95%) represents. It does not mean that 95% of the sample data lies within the confidence interval. Instead, it implies that if we were to take multiple samples and compute the interval for each, 95% of these intervals would contain the true population parameter.

Mistake 2: Using Confidence Intervals for Predictions: Another common error is using confidence intervals to predict future observations. Confidence intervals are based on sample data and are used to estimate the population parameter, not to predict future outcomes.

Mistake 3: Neglecting Assumptions Underlying Confidence Interval Calculations: The accuracy of a confidence interval depends on certain assumptions, such as the data being normally distributed or the sample being randomly selected. Ignoring these assumptions can lead to inaccurate intervals and misleading conclusions.

Conclusion

In conclusion, this comprehensive exploration into the world of confidence intervals and the utilization of the confidence interval calculator underscores the profound significance and versatility of these statistical tools in various domains of data analysis.

Reiterating Key Insights:

Confidence Intervals as a Statistical Compass: We have seen how confidence intervals provide a range of values and a reflection of the reliability and precision inherent in data estimates. They serve as a critical compass in navigating the uncertainty of statistical analysis, offering a balanced view between the known and the unknown.

The Calculator as a Bridge to Understanding: The confidence interval calculator, highlighted in this guide, stands out as a bridge, connecting intricate statistical computations with the simplicity needed for wider accessibility. It empowers both experienced statisticians and beginners, facilitating accurate and efficient analysis without the intimidating complexity of manual calculations.

Applications Across Diverse Fields: The practical applications of confidence intervals, from scientific research to market analysis, policy-making, quality control, and financial analysis, demonstrate their ubiquitous relevance. They are indispensable tools in making informed, data-driven decisions across numerous sectors.

Navigating Misconceptions and Mistakes: Addressing common misconceptions and mistakes associated with confidence intervals is crucial. It ensures that these tools are used correctly and effectively, enhancing the integrity and reliability of statistical analyses.

Frequently Asked Questions (FAQs)

Q1: What is a Confidence Interval? It’s a range of values derived from the data, within which we can say with a certain level of confidence the true value lies.

Q2: How Does the Confidence Interval Calculator Work? The calculator uses your data’s mean, standard deviation, and sample size to compute the interval with a chosen confidence level.

Q3: Why is the Confidence Interval Important in Statistics? It offers a measure of reliability for an estimate, helping in understanding the precision of sample estimates.

Q4: What Does a 95% Confidence Interval Mean? If the same study were repeated multiple times, 95% of the calculated intervals would contain the true population parameter.

Q5: How Does Sample Size Affect the Confidence Interval? Generally, a larger sample size results in a narrower confidence interval, offering more precision.

Q6: Can Confidence Intervals Predict Future Outcomes? No, they reflect the reliability of current data and are not predictive of future observations.

Q7: What is the Difference Between Confidence Interval and Confidence Level? The interval is the range of values, whereas the confidence level indicates how often the true value will fall within this range.

Q8: Are Confidence Intervals Only Used in Scientific Research? No, they are also used in market research, quality control, and other areas requiring statistical analysis.

Q9: Can a Confidence Interval Include Negative Numbers? Yes, it depends on the data. Negative intervals can occur, particularly with differences or changes.

Q10: How Do I Choose the Right Confidence Level for My Analysis? It depends on your tolerance for risk; higher confidence levels reduce risk but widen the interval.